Part II. ACT DIAGNOSTIC ASSESSMENT

2. MATHEMATICS TEST

60 Minutes – 60 Questions

DIRECTIONS: Solve each of the problems in the time allowed, then fill in the corresponding bubble on your answer sheet. Do not spend too much time on any one problem; skip the more difficult problems and go back to them later. You may use a calculator on this test. For this test you should assume that figures are NOT necessarily drawn to scale, that all geometric figures lie in a plane, and that the word line is used to indicate a straight line.

1.   If 4x – 9 = 11, then x = ?

A.  5

B.  6

C.  6.5

D.  9

E.  16

2.   Consider the following 2 logical statements:

If the length of  is 4, then the length of  is 7.

The length of  is NOT 7.

If these statements are both true, then the length of:

F.   is NOT 4

G.   is 7

H.   is 4

 J.   is NOT 4

K.   is 7

3.   If 60% of a given number is 9, then what is 25% of the given number?

A.  0.66

B.  1.75

C.  2.33

D.  3.75

E.  6.50

4.   Vehicle A averages 16 miles per gallon of gasoline, and Vehicle B averages 35 miles per gallon of gasoline. At these rates, how many more gallons of gasoline does Vehicle A need than Vehicle B to make a 1,120-mile trip?

F.  32

G.  38

H.  46

 J.  63

K.  70

5.   The five consecutive integers below add up to 390.

                  x – 3

                  x – 2

                  x – 1

                  x

                  x + 1

What is the value of x?

A.  79

B.  80

C.  81

D.  82

E.  83

6.   If P = 5a and Q = 3b – 2a, then what is the value of P – Q?

F.  7a + 3b

G.  3a + 3b

H.  7a – 3b

 J.  3a – 3b

K.  5a – 3b

7.   In the figure below, l₁ is parallel to l₂, l₃ is parallel to l₄, and the lines intersect as shown. What is the measure of angle z?

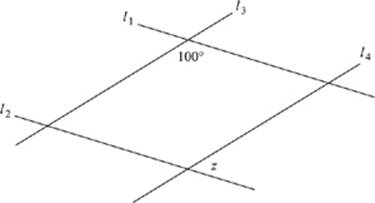
A.  40°

B.  50°

C.  60°

D.  70°

E.  80°

8.   If x = 2, then –(x²) + 4x – 3 = ?

F.  9

G.  5

H.  1

 J.  –1

K.  –7

9.   The average of 8 numbers is 6.5. If each of the numbers is decreased by 3, what is the average of the 8 new numbers?

A.  0.0

B.  3.5

C.  4.0

D.  7.5

E.  9.5

10.   The expression 5a + 5b is equivalent to which of the following?

F.  5(a – b)

G.  10(a + b)

H.  5ab

 J.  5(a + b)

K.  10ab

11.   An interior designer charges $25 for each hour that she works on a project, plus a flat $40 project fee. Approximately how many hours of work are included in a $375 bill for a project?

A.  4.5

B.  5.8

C.  9.4

D.  13.4

E.  15.0

12.   If , what is the largest possible value for x?

F.  

G.  4

H.  16

 J.  24

K.  32

13.   On the clock shown below, what is the number of degrees that the hour hand of the clock moves from 1:00 P.M. to 8:00 P.M.?

A.  70°

B.  150°

C.  210°

D.  270°

E.  300°

14.   In the standard (x, y) coordinate plane below, PQRS is a parallelogram. Points P, Q, and S are located on the axes as shown. Which of the following could be the coordinates of point R?

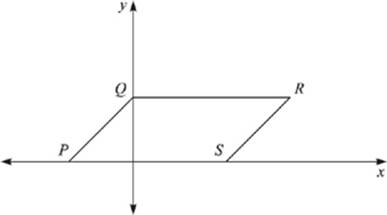
F.  (0, 4)

G.  (4, 0)

H.  (–3, 0)

 J.  (6, 4)

K.  (6, –4)

15.   Which of the following is a factored form of 3x³y³ + 3xy?

A.  3xy(x²y² + 1)

B.  3(3x²y²)

C.  (3x + 3y)(3x + 3y)

D.  3x²y²(xy)

E.  3x(x²y² + 3)

16.   A classroom has (r + s) rows of seats and t seats in each row. Which of the following is an expression for the number of seats in the entire classroom?

F.  rst

G.  (rs) + (rt)

H.  t + (rs)

 J.  r + s + t

K.  (rt) + (st)

17.   If 20% of x equals 16, then x = ?

A.  2

B.  3.2

C.  32

D.  80

E.  800

18.   In a 5-kilometer race, runners recorded times (in minutes : seconds) of 24: 04, 22: 45, 19: 53, and 21: 33. What is the difference between the slowest time and the fastest time?

F.  2:23

G.  2:45

H.  4:11

 J.  4:51

K.  5:38

19.   You are standing in line at the cash register to pay for a watch priced at $12.99. A sales tax of 6% of the $12.99 will be added (rounded to the nearest cent) to the price of the watch. You have 15 one-dollar bills, but how much will you need in coins if you want to have exact change ready?

A.  $0.23

B.  $0.33

C.  $0.53

D.  $0.67

E.  $0.77

20.   For which nonnegative value of x is the expression  undefined?

F.  0

G.  4

H.  16

 J.  32

K.  256

21.   What is the smallest integer greater than 

A.  3

B.  9

C.  10

D.  11

E.  50

22.   Two strips of tape are to be used to seal a box, as shown below. Both strips must go completely around the box. What is the minimum length of tape, in centimeters (cm), required to seal the box?

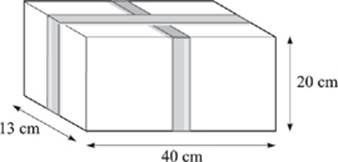
F.  73 cm

G.  112 cm

H.  120 cm

 J.  146 cm

K.  186 cm

23.   Kahla owns 2 bicycle shops (A and B). She stocks 3 brands of biking shorts (X, Y, and Z) in each store. The matrices below show the numbers of each type of biking shorts in each store and the cost for each type of biking short. The value of Kahla’s biking short inventory is computed using the costs listed. What is the total value of the biking short inventory for Kahla’s 2 stores?

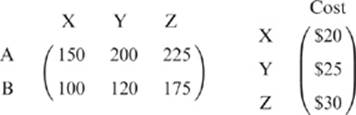
A.  $25,000

B.  $20,000

C.  $13,000

D.  $14,750

E.  $10,250

24.   Which of the following gives all the solutions of x² + 2x = 8?

F.  4 and –2

G.  –4 and 2

H.  –8 and 1

 J.  –4 only

K.  –8 only

25.   If (f + g)² = 81 and fg = 20, then f² + g² = ?

A.  1

B.  9

C.  41

D.  81

E.  100

26.   If, for all x, (x^4a –3)² = x¹⁰, then a = ?

F.  

G.  1

H.  

 J.  2

K.  

27.   For the complex number i such that i² = 1, what is the value of i⁶ + 3i⁴?

A.  –2

B.  –1

C.  0

D.  1

E.  2

28.   In the (x, y) coordinate plane, what is the y-intercept of the line 5x – 4y = 7?

F.  –4

G.  

H.  

 J.  

K.  7

29.   In the (x, y) coordinate plane, what is the radius of the circle with the equation (x + 3)² + (y – 2)² = 10?

A.  2

B.  3

C.  

D.  

E.  10

30.   In the right triangle pictured below, l, m, and n are the lengths of its sides. What is the value of sin β?

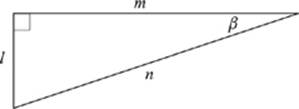
F.  

G.  

H.  

 J.  

K.  

31.   For all nonzero a and b, 

A.  2a⁴b²

B.  –2a²b²

C.  

D.  

E.  –2a⁴b²

32.   In the figure below, 3 parallel lines are crossed by 2 transversals, as shown. The points of intersection and some distances, in inches, are labeled. What is the length, in inches, of x?

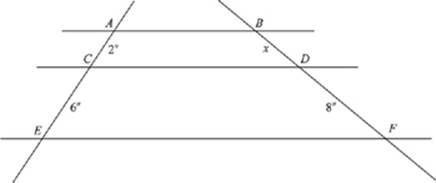
F.  2

G.  

H.  3

 J.  

K.  4

33.   The figure below shows square ABCD and also shows the circle centered at D with radii  and  If the perimeter of the square is 28 units, what is the area of the circle, in square units?

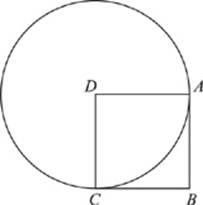
A.  7π

B.  14π

C.  28π

D.  49π

E.  56π

34.   Which of the following logical statements identifies the same set as the graph shown below?

F.  x ≤ 2 or x ≥ 4

G.  x ≤ 2 and x ≥ 4

H.  x < 2 or x > 4

 J.  x ≤ 2 and x > 4

K.  x ≤ 2 or x > 4

35.   A right circular cylinder is shown below, with dimensions given in inches. What is the total surface area of this cylinder, in square inches?
(Note: The total surface area of a cylinder is given by 2πr² +2πrh, where r is the radius and h is the height.)

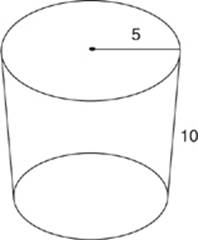
A.  50π

B.  100π

C.  120π

D.  150π

E.  200π

36.   If x and y are real and , then what must be true of the value of y?

F.  y must be negative

G.  y must be positive

H.  y must equal 4

 J.  y must equal 

K.  y may have any value

37.   If c is a positive integer that divides evenly into both 64 and 96 but divides evenly into neither 16 nor 20, what should you get when you add the digits in c?

A.  3

B.  5

C.  7

D.  8

E.  10

38.   What is the slope of any line parallel to the y-axis in the (x, y) coordinate plane?

F.  –1

G.  0

H.  1

 J.  Undefined

K.  Cannot be determined from the given information

39.   If point J has a nonzero x-coordinate and a nonzero y-coordinate and the coordinates have the same sign, then point J must be located in which of the 4 quadrants shown below?

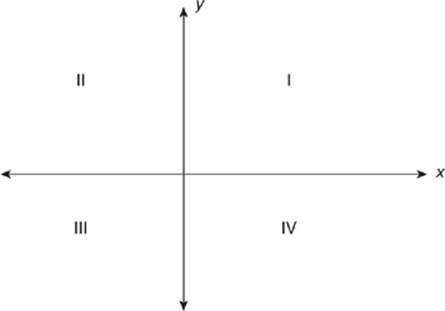
A.  I only

B.  II only

C.  III only

D.  I or III only

E.  II or IV only

40.   The 2 triangles in the rectangle below share a common side. What is sin(a – b)?

(Note: sin(a – b) = sin a cos b – cos a sin b for all a and b.)

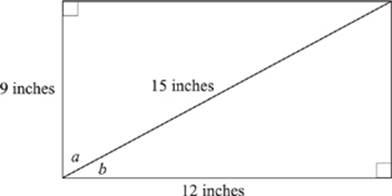
F.  

G.  

H.  

 J.  1

K.  

41.   Which of the following represents 1/100 of 10%?

A.  1.0

B.  0.1

C.  0.01

D.  0.001

E.  0.00

42.   Which of the following calculations will yield an odd integer for any integer n?

F.  4n²

G.  3n² + 1

H.  6n²

 J.  n² – 1

K.  4n² – 1

43.   In triangle ABC, the measure of ∠ A is 60° and the measure of ∠B is 30°. If  is 8 units long, what is the area, in square units, of triangle ABC?

A.  4

B.  

C.  8

D.  

E.  

44.   In the figure below, all distances are in feet and all angles are right angles. A straight line drawn from point W to point Z would be how long, in feet?

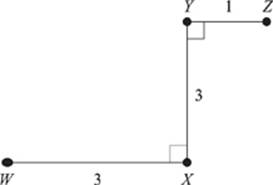
F.  1.5

G.  2

H.  4.66

 J.  5

K.  7.23

45.   A certain rectangle is 4 times as long as it is wide. Suppose the length and width are tripled. The area of the second rectangle is how many times as large as the area of the first?

A.  3

B.  4

C.  9

D.  12

E.  16

46.   For what value of b would the following system of equations have an infinite number of solutions?

3x + 4y = 14

6x + 8y = 7b

F.  2

G.  4

H.  7

 J.  14

K.  28

47.   If log₃ x = 2, then x = ?

A.  

B.  3

C.  6

D.  9

E.  18²

48.   When measured from a point on the ground that is a certain distance from the base of a telephone pole, the angle of elevation to the top of the telephone pole is 37°, as shown below. The height of the telephone pole is 24 feet. What is the distance, in feet, to the telephone pole?

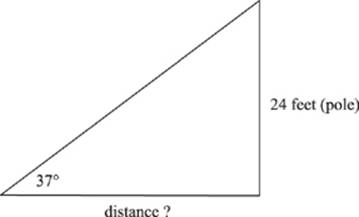
F.  24 tan 37°

G.  24 sin 37°

H.  24 cos 37°

 J.  24 sec 37°

K.  24 cot 37°

49.   In the parallelogram below, lengths are given in inches. What is the area of the parallelogram, in square inches?

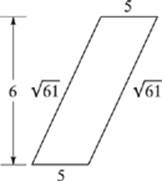
A.  15

B.  

C.  30

D.  

E.  

50.   Points A, B, and C are 3 distinct points that lie on the same line. If the length of  is 12 meters and the length of  is 15 meters, then what are all the possible lengths, in meters, for 

F.  3 only

G.  27 only

H.  3 and 27 only

 J.  Any number less than 27 or greater than 3

K.  Any number greater than 27 or less than 3

51.   If the right triangle and the rectangle in the figure below have the same area, and indicated lengths are given in centimeters, what is a expressed in terms of b?

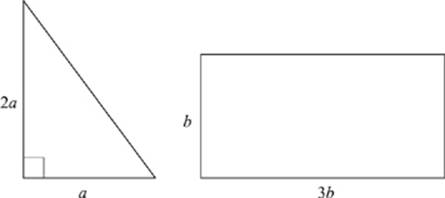
A.  b

B.  

C.  

D.  

E.  

52.   If 6a⁴b³ < 0, then which of the following CANNOT be true?

F.  b < 0

G.  b > 0

H.  a = b

 J.  a < 0

K.  a > 0

53.   The 1st and 2nd terms of a geometric sequence are p and sp, in that order. What is the 734th term of the sequence?

A.  (sp)⁷³³

B.  (sp)⁷³⁴

C.  s⁷³³p

D.  s⁷³⁴p

E.  sp⁷³³

54.   If a system of 2 linear equations in 2 variables has NO solution, and 1 of the equations is graphed in the (x, y) coordinate plane below, which of the following could be the equation of the other line?

F.  y = –2

G.  y = 4x + 2

H.  y = 2x –3

 J.  y = 4x + 2

K.  y = 4x –3

55.   If 0° ≤ x ≤ 90° and tan , then cos x = ?

A.  

B.  

C.  

D.  

E.  

56.   If L, M, and N are real numbers, and if LMN = 1, which of the following conditions must be true?

F.  

G.  L, M, and N must all be positive

H.  Either L = 1, M = 1, or N = 1

 J.  Either L = 0, M = 0, or N = 0

K.  Either L < 1, M < 1, or N < 1

57.   Which of the following is a rational number?

A.  

B.  

C.  

D.  

E.  

58.   In order to clean her aquarium, Rana must remove half of the water. The aquarium measures 24 inches long, 12 inches wide, and 10 inches deep. The aquarium is currently completely full. What volume of water, in cubic inches, must Rana remove?

F.  288

G.  960

H.  1,440

 J.  2,880

K.  5,760

59.   In the figure below, line p has the equation y = 3x. Line q is below p, as shown, and q is parallel to p. Which of the following is an equation for q?

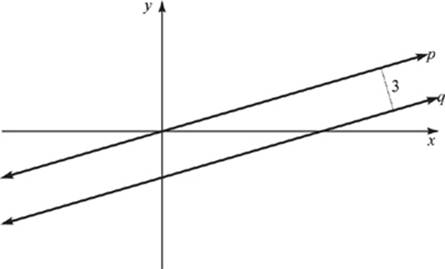
A.  y = x – 3

B.  

C.  

D.  y = 3x – 3

E.  y = 3x + 3

60.   What is the smallest possible value for the product of 2 real numbers that differ by 12?

F.  – 36

G.  – 27

H.  – 11

 J.  0

K.  13

END OF THE MATHEMATICS TEST
STOP! IF YOU HAVE TIME LEFT OVER, CHECK YOUR WORK ON THIS SECTION ONLY.