MCAT General Chemistry Review
Chapter 4: Compounds and Stoichiometry
4.1 Molecules and Moles
A molecule is a combination of two or more atoms held together by covalent bonds. Molecules are the smallest units of compounds that display their identifying properties. Molecules can be composed of two or more atoms of the same element (such as N2 and O2) or may be composed of atoms of different elements, as in CO2 (carbon dioxide), SOCl2 (thionyl chloride), and C6H5CHO (benzaldehyde). Because reactions usually involve a very large number of molecules—far too many to count individually—we usually measure amounts of compounds in terms of moles or grams, using molar mass to interconvert between these units.
Ionic compounds do not form true molecules because of the way in which the oppositely charged ions arrange themselves in the solid state. As solids, they can be considered as nearly infinite three-dimensional arrays of the charged particles that comprise the compound. As described in Chapter 3 of MCAT General Chemistry Review, solid NaCl is a coordinated lattice in which each of the Na+ ions is surrounded by Cl– ions and each of the Cl– ions is surrounded by Na+ ions. This makes it rather difficult to clearly define a sodium chloride molecule, and the term formula unit, representing the empirical formula of the compound, is used instead. Because no molecule actually exists, molecular weight becomes meaningless, and the term formula weight is used instead.
BRIDGE
Ionic compounds form from combinations of elements with large electronegativity differences, such as sodium and chlorine. Molecular compounds form from elements of similar electronegativity, such as carbon with oxygen. The difference between ionic and covalent bonds is discussed in Chapter 3 of MCAT General Chemistry Review.
MOLECULAR WEIGHT
Remember that the term atomic weight is a misnomer because it is actually a weighted average of the masses of the naturally occurring isotopes of an element, not their weights. The same applies here to our discussion of molecular weight. Molecular weight, then, is simply the sum of the atomic weights of all the atoms in a molecule, and its units are atomic mass units (amu) per molecule. Similarly, the formula weight of an ionic compound is found by adding up the atomic weights of the constituent ions according to its empirical formula, and its units are also amu per molecule.
Example:
What is the molecular weight of SOCl2?
Solution:
To find the molecular weight of SOCl2, add together the atomic weights of each of the atoms.
|
1 S: 1 × 32.1 amu |
= |
32.1 amu |
|
1 O: 1 × 16.0 amu |
= |
16.0 amu |
|
2 Cl: 2 × 35.5 amu |
= |
71.0 amu |
|
Total molecular weight |
= |
119.1 amu per molecule |
MOLE
A mole is a quantity of any substance (atoms, molecules, dollar bills, kittens—anything) equal to the number of particles that are found in 12 grams of carbon-12 (612C). This number of particles is defined as Avogadro’s number (NA), 6.022 × 1023 mol–1. One mole of a compound has a mass in grams equal to the molecular or formula weight of the compound in amu. For example, one molecule of H2CO3 (carbonic acid) has a mass of 62 amu; one mole of the compound has a mass of 62 grams. The mass of one mole of a compound is called its molar mass and is usually expressed in ![]() The term molecular weight is sometimes used incorrectly to imply molar mass; remember, molecular weight is measured in
The term molecular weight is sometimes used incorrectly to imply molar mass; remember, molecular weight is measured in ![]() , not
, not ![]()
KEY CONCEPT
Remember that Avogadro’s number (and the mole) are just units of convenience, like the dozen is a convenient unit for eggs.
The formula for determining the number of moles of a substance sample is:
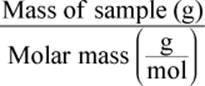
Equation 4.1
This equation is often used in stoichiometry and titration problems.
Example:
How many moles are in 9.53 g of MgCl2?
Solution:
First, find the molar mass of MgCl2.
![]()
Now, solve for the number of moles.

EQUIVALENT WEIGHT
Equivalent weight and the related concept of equivalents are a source of confusion for many students. Part of the problem may be the context in which equivalents and equivalent weights are usually discussed: acid–base reactions, oxidation–reduction reactions, and precipitation reactions, all three of which can be sources of confusion and anxiety on their own. Therefore, let’s start with a more basic discussion of equivalents.
Often, certain elements or compounds can act more potently than others in performing certain reactions. For example, one mole of HCl has the ability to donate one mole of hydrogen ions (H+) in solution, but one mole of H2SO4 has the ability to donate two moles of hydrogen ions, and one mole of H3PO4 has the ability to donate three moles of hydrogen ions. To gather one mole of hydrogen ions for a particular acid–base reaction, we could use one mole of HCl, a half-mole of H2SO4, or one-third of a mole of H3PO4. Or, consider the difference between Na and Mg: One mole of sodium has the ability to donate one mole of electrons, while one mole of magnesium has the ability to donate two moles of electrons. This provides context for the concept of equivalents: How many moles of the thing we are interested in (protons, hydroxide ions, electrons, or ions) will one mole of a given compound produce? Sodium will donate one mole of electrons (one equivalent), but magnesium will donate two moles of electrons (two equivalents).
BRIDGE
The idea of equivalents is related to the concept of normality, which is explained in the discussion of acids and bases in Chapter 10 of MCAT General Chemistry Review.
So far, this discussion has been focused on the mole-to-mole relationship between, say, an acid compound and the hydrogen ions it donates. However, sometimes we need to work in units of mass rather than moles. Just as one mole of HCl will donate one mole of hydrogen ions, a certain mass of HCl (about 36.5 g) will also donate one equivalent of hydrogen ions. This amount of a compound, measured in grams, that produces one equivalent of the particle of interest is called the gram equivalent weight and can be calculated from:
![]()
Equation 4.2
where n is the number of particles of interest produced or consumed per molecule of the compound in the reaction. For example, one would need 31 grams of H2CO3 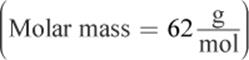 ) to produce one equivalent of hydrogen ions because each molecule of H2CO3 can donate two hydrogen ions (n = 2). Simply put, the equivalent weight of a compound is the mass that provides one mole of the particle of interest.
) to produce one equivalent of hydrogen ions because each molecule of H2CO3 can donate two hydrogen ions (n = 2). Simply put, the equivalent weight of a compound is the mass that provides one mole of the particle of interest.
MCAT EXPERTISE
Whenever confronting a stoichiometry problem, always look for normality by identifying an equivalent unit (protons, hydroxide ions, electrons, ions) and then multiplying it by the number of moles or molar concentration to find the normal concentration.
If the amount of a compound in a reaction is known and we need to determine how many equivalents are present, use the equation:
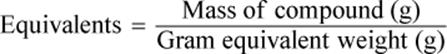
Equation 4.3
Finally, we can now introduce the measurement of normality. Normality (N) is a measure of concentration, given in the units ![]() It is most commonly used for hydrogen ion concentration. Thus, a 1 N solution of acid contains a concentration of hydrogen ions equal to 1 mole per liter; a 2 N solution of acid contains a concentration of hydrogen ions equal to 2 moles per liter. The actual concentration of the acidic compound may be the same or different from the normality because different compounds are able to donate different numbers of hydrogen ions. In a 1 NHCl solution, the molarity of HCl is 1 M because HCl is a monoprotic acid; in a 1 N H2CO3 solution, the molarity of H2CO3 is 0.5 M because H2CO3 is a diprotic acid. Note that because normality calculations always assume that a reaction will proceed to completion; while carbonic acid does not fully dissociate in solution, it can be reacted with enough base for each molecule to give up both of its protons. The conversion from normality to molarity of a given solute is:
It is most commonly used for hydrogen ion concentration. Thus, a 1 N solution of acid contains a concentration of hydrogen ions equal to 1 mole per liter; a 2 N solution of acid contains a concentration of hydrogen ions equal to 2 moles per liter. The actual concentration of the acidic compound may be the same or different from the normality because different compounds are able to donate different numbers of hydrogen ions. In a 1 NHCl solution, the molarity of HCl is 1 M because HCl is a monoprotic acid; in a 1 N H2CO3 solution, the molarity of H2CO3 is 0.5 M because H2CO3 is a diprotic acid. Note that because normality calculations always assume that a reaction will proceed to completion; while carbonic acid does not fully dissociate in solution, it can be reacted with enough base for each molecule to give up both of its protons. The conversion from normality to molarity of a given solute is:
![]()
Equation 4.4
where n is the number of protons, hydroxide ions, electrons, or ions produced or consumed by the solute.
Figure 4.1 shows the titration of the diprotic acid H2CO3 with a base. The x-axis indicates that two equivalents of base are needed to neutralize both protons of this acid.
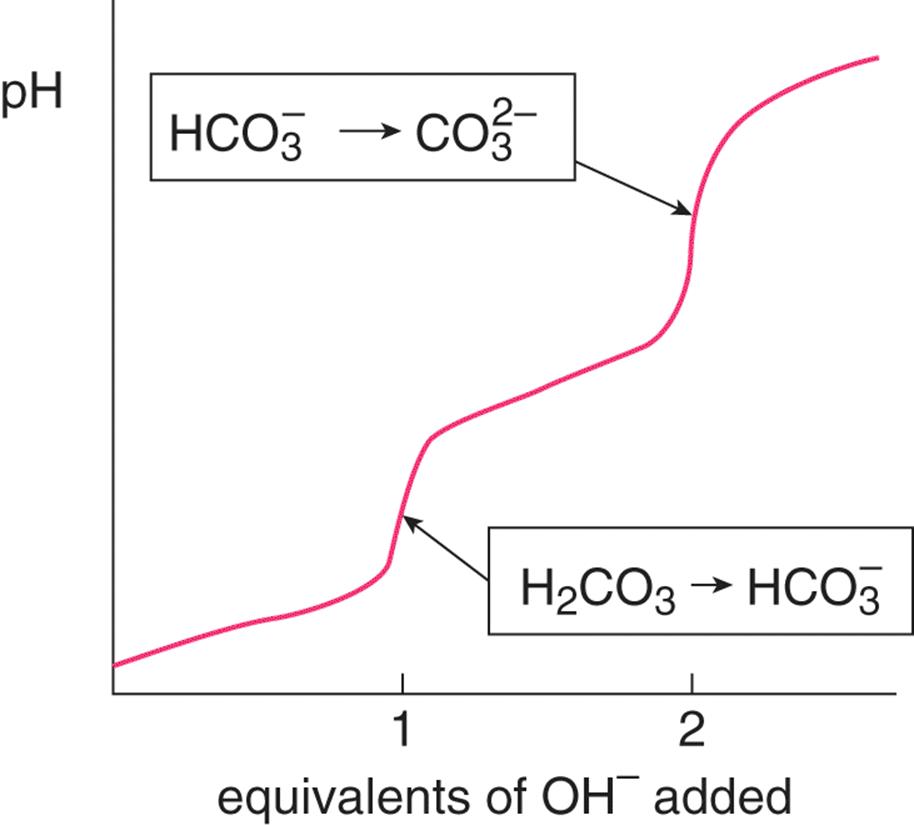 Figure 4.1. Titration of Carbonic Acid with a Base Carbonic acid is diprotic, so two equivalents of base are required to neutralize both protons of the acid.
Figure 4.1. Titration of Carbonic Acid with a Base Carbonic acid is diprotic, so two equivalents of base are required to neutralize both protons of the acid.
There is a real benefit to working with equivalents and normality because it allows a direct comparison of the quantities of the entity we are most interested in. In an acid–base reaction, we care about the hydrogen or hydroxide ions; where the ions come from is not really the primary concern. It is convenient to be able to say that one equivalent of acid (hydrogen ions) will neutralize one equivalent of base (hydroxide ions), but the same could not necessarily be said if we were dealing with moles of acidic compounds and moles of basic compounds. For example, one mole of HCl will not completely neutralize one mole of Ca(OH)2 because one mole of HCl will donate one equivalent of acid, but Ca(OH)2 will donate two equivalents of base.
Example:
What is the gram equivalent weight of sulfuric acid?
Solution:
First, find the molar mass of H2SO4.
![]()
Next, identify the equivalents: protons (H+), because these are transferred in acid–base reactions. The number of protons in sulfuric acid (n) is 2.
Now, calculate the gram equivalent weight.
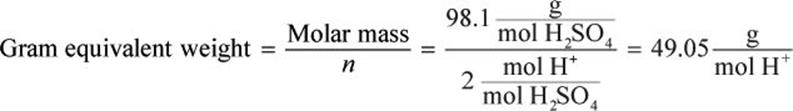
KEY CONCEPT
In acid–base chemistry, the gram equivalent weight of an acid represents the mass that yields one mole of protons, or one mole of hydroxide ions if a base.
Example:
What is the normality of a 2 M Mg(OH)2 solution?
Solution:
First, identify the number of equivalents (n). There are two hydroxide ions (OH–), which is the most likely equivalent of interest because magnesium hydroxide is a base.
Then, calculate the normality.

MCAT Concept Check 4.1:
Before you move on, assess your understanding of the material with these questions.
1. Calculate the molar masses of the following substances:
· NaBr:
· CuCl2:
· C6H12O6:
2. Calculate the number of moles in 100 g of each of the following substances:
· NaBr:
· CuCl2:
· C6H12O6:
3. How do the number of molecules in 18 g of H2O compare to the number of formula units in 58.5 g of NaCl?
4. Determine the normality of the following solutions: (Note: The species of interest is H+.)
· 0.25 M H3PO4:
· 95 g PO43− in 100 mL solution: