Homework Helpers: Physics

1 Kinematics

Think about some of the experiences that you have had with falling objects. Have you ever dropped an object, such as a glass, and been relieved when it didn”t break? Did you ever drop water balloons out of a high window? Did you ever make a connection between how high a baseball flies, and how much it stings your hand when you catch it?

Why is it that when you throw a baseball a few feet into the air you can catch it with your bare hand and not feel a sting, but when a baseball falls from a much higher point, like when someone hits a pop fly, the ball hits your hand hard enough to hurt? The answer, of course, is that the ball is falling faster when it has fallen a greater distance. This is clear evidence that objects accelerate (speed up) as they fall.

Can you imagine what the world would be like if objects didn”t accelerate as they fell? If objects maintained a constant velocity as they fell, then jumping off a 50-story building would be the same as jumping down from the curb! Dropping a glass from an inch above the floor would be the same as dropping one off a cliff! But, of course, falling objects do accelerate.

Why do objects accelerate as they fall? You will learn more about this in our next chapter, as we study Newton”s second law of motion. The force of gravity causes objects to accelerate, but we said that we wouldn”t discuss forces in this chapter. We won”t discuss why the objects accelerate yet, but we will discuss how they accelerate.

When objects are made to fall close to the surface of Earth, where the only unbalanced force acting on them is gravity, we say that they are “falling freely” or experiencing free fall. Gravity will cause such objects to accelerate at a constant rate of 9.81 m/s². This rate of acceleration is a constant (near the surface of Earth) called “the acceleration due to gravity,” and is given the symbol g. Be careful not to confuse the symbol for the acceleration due to gravity with the symbol for grams.

Notice that there is a difference between constant velocity and constant acceleration. If an object has a constant velocity, it means that it doesn”t speed up, slow down, or change direction. An object that has a constant nonzero acceleration does speed up, slow down, and/or change direction. When we say that an object is accelerating at a rate of 9.81 m/s², we mean that every second that it is falling, its velocity changes by 9.81 m/s. If you throw a ball upward, it will experience this acceleration during its entire flight. On the way up, its velocity is decreasing at a rate of 9.81 m/s every second, until it reaches a velocity of zero. After that, it will begin to come back down, and its velocity will be increasing by 9.81 m/s every second. It is very important to remember that its acceleration doesn”t change during the entire flight (g is a constant) but its velocity certainly does.

We have spent some time in this chapter discussing the idea of using signs for direction. It is a fairly standard practice for physics students and teachers to designate “upwards” as positive and “downward” as negative. Using this sign convention, that means that we will use the value of –9.81 m/s² for g.

The Acceleration Due to Gravity Close to the Surface of Earth

g = –9.81 m/s²

It is important for you to remember that g, the acceleration due to gravity, is just a specific acceleration. This means that you can use any of the formulas for uniform acceleration for free-fall problems. You just substitute g for a. Some students and teachers prefer to rewrite the formulas to include the g, as shown here, to remind them to use the value of –9.81 m/s² for the acceleration.

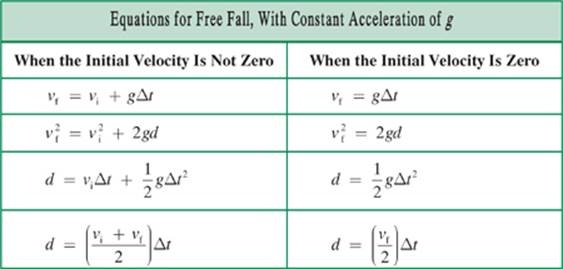
Of course, there is really no need to memorize or even rewrite a new set of equations. Substituting g for a is simply done to remind us that the acceleration for each of these problems is a constant, which we can count among our “given” quantities. You will find that the problems that we cover in this lesson are really just special examples of the uniform equations that we covered in Lesson 1–4. The only difference is that the acceleration is not usually specifically stated in these problems, because it is a constant.

Let”s try some examples.

Example 1

A boy drops a rock into well and times how long it takes for him to hear it strike the water below. If the rock takes 2.1 seconds to hit the water, how deep is the well?

Ah, the classic “boy drops a rock in a well problem.” Despite the fact that many of today”s physics students may never see a well, this classic never seems to go out of style. One key to solving these problems is proper interpretation. When the problem states that a boy “drops” a rock it implies that the rock is starting from rest, with an initial velocity of zero. If the boy “threw” the rock, it would be a different story, and an initial velocity would be included in the problem. The other key is to remember that once the boy releases the rock, the only unbalanced force acting on it (when we ignore friction) is the force of gravity. Because this is an example of an object in free fall, the acceleration that it experiences will be equal to g, –9.81 m/s². Given all of this, can you solve the problem before checking my answer?

Given: v_i = 0.0 m/s Δt – 2.1s g = –9.81m/s²

Find: d

It might surprise you to find that the displacement has a negative value, but it shouldn”t be such a shock. Remember: We have decided to designate “downward” as the negative direction, so the negative sign in our answer tells us that the rock fell downward, rather than upward. Isn”t it interesting that you can use this technique in real life to determine the height or depth of something? The physics that you learn has all kinds of practical applications

Let”s try an example with an object going upward. Read the question and try the problem on your own before checking my answer.

Example 2

An archer points her bow straight upward and fires an arrow with an initial velocity of 22.0 m/s. What is the maximum height that the arrow will reach?

For this type of problem, you need to remember that the arrow will have a positive (upward) initial velocity, but a negative (downward) acceleration. This means that the arrow will go slower and slower as it goes higher and higher. Eventually, the velocity of the arrow will become zero, at the instant that it reaches its maximum height (max d_y), or displacement. After that, the arrow starts to fall back down, accelerating along the way. So, that archer best get out of the way! The key to this type of problem is to use the value of zero for the final velocity, even though it is only the final velocity for the upward motion of the arrow. Sometimes I will call it v_f (at max d_y) to remind myself that the arrow still needs to fall back down.

Given: v_i = 22.0m/s v_f(at maxd_y) = 0.00m/s  g = –9.81m/s²

Find: d

Many problems that you will encounter in physics will have multiple parts. For example, we can use the problem from Example 2 and find the time that it takes the arrow to reach its maximum height. You might be tempted to use the value for d, which we just calculated, as one of the givens for the second part of the problem, but I am going to caution you not to for two reasons. First, the value that we have for d has been rounded already, and second, it is possible that we might have made an error in our calculations. Whenever possible, try to avoid using the answers from one part of a problem to solve the next part of a problem. If you can select a formula that allows you to solve the second part without relying on prior calculations, it is advisable to do so. You won”t always have this option, but when you do, I suggest that you take it.

Example 3

An archer points her bow straight upward and fires an arrow with an initial velocity of 22.0 m/s. How many seconds will it take the arrow to reach its maximum height?

Given: v_i = 22.0m/s v_f(at maxdy) = 0.00m/s g = –9.81m/s²

Find: Δt

Keep in mind that the change in time that we just solved for only represents the amount of time that it takes for the arrow to reach its maximum height. Because the acceleration on the object is constant, it will require the same amount of time to fall back down to its original height (the height from which it was fired). If you were asked to determine the total time of flight, you would need to multiply the change of time that we calculated by 2, to take into account the time it takes for the arrow to fall back down. Of course, in a slightly harder type of problem, you might be asked to take the height of the archer into account.

For our last example, let”s calculate the final velocity of a falling object that has been thrown downward with an initial velocity in the negative direction. Although a force is initially exerted on such an object, after the object leaves the hand it is influenced only by gravity (when we ignore air resistance) so this, too, is an example of free fall.

Example 4

A child throws a rock with an initial velocity of –8.00 m/s, straight downward, from a window to strike the ground 30.0 m below. With what velocity will the rock strike the ground?

Given: v_i = –8.00m/s d = –30.0 m g = –9.81m/s²

Find: v_f

Which rounds to –25.5 m/s. Note: We added the negative sign to our numerical answer to indicate that the direction of the final velocity is in the downward direction.

Lesson 1–5 Review

1. _______________ is the condition of an object that is falling with uniform acceleration caused by gravity.

2. When a baseball player hits a pop fly, what is the velocity and the acceleration of the ball when it reaches its highest point?

3. If a bullet were fired straight up with an initial velocity of 80.0 m/s, how high would it go? (Ignore air resistance.)