SAT Subject Test Physics (2012)
PART III. PHYSICS TOPIC REVIEW
Chapter 10. HEAT AND TEMPERATURE
Energy can be used to describe motion on macroscopic scales in terms of motion and work. It can also be used on a microscopic level to describe the properties of matter. SAT Physics will ask several different kinds of questions involving the motion and energy of particles of matter. In this chapter, you will review some of those topics, including heat, temperature, and phase changes.
Kinetic Theory
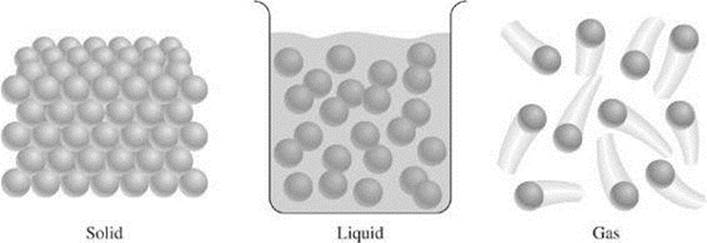
Matter is anything that has mass and takes up space. The kinetic theory describes the physical properties of matter in terms of the motion of its particles, which can be molecules, atoms, or ions. According to the kinetic theory, all matter is made up of tiny particles that are in constant motion. In a gas, the particles move rapidly in all directions. In a liquid, the particles are held closer together and can only slide past one another. In a solid, the particles are arranged tightly together in a regular pattern and only vibrate in place. The kinetic theory, therefore, can be used to explain the differences among the states of matter. More on this topic will be covered later in this chapter as well as in the next chapter.
Thermal Energy
Objects have kinetic energy as a result of their motion. Particles of matter, therefore, have kinetic energy because they are in motion. Like any other object, the kinetic energy of a particle of matter depends on the mass and velocity of the particle.
![]()
The total kinetic energy of all the particles in a sample of matter is its thermal energy. Like other forms of energy, thermal energy is measured in joules.
Thermal energy flows from warmer substances to cooler substances. The transfer of thermal energy from a warmer substance to a cooler substance is known as heat. When a substance absorbs heat, its particles move faster and farther apart. When a substance loses heat, its particles slow down and remain closer together.
Keep in mind that you may come across a related term, internal energy. Thermal energy is a portion of internal energy. In a sample of matter, internal energy is the total amount of energy of the particles and includes potential energy in addition to kinetic energy.
Temperature
The average kinetic energy of the molecules in a sample of matter is its temperature. When a sample is heated, therefore, its temperature can rise because the motion of its particles increases. When a sample cools, the particles slow down thereby decreasing the average kinetic energy and temperature. Simply put, temperature is a measure of how hot or cold a substance is.
Note that temperature describes an average, whereas thermal energy describes a total. Therefore, two samples, such as a pot of hot tea and a cup of hot tea, can have the same temperature but different amounts of thermal energy.
Temperature is measured with a thermometer. The SI unit of temperature is the kelvin (K), measured on the Kelvin scale. However, temperature is more commonly measured on the Celsius scale (°C). The two scales are closely related. At standard pressure, water freezes at 0°C and boils at 100°C. The Kelvin scale increases by the same increments as the Celsius scale. The Kelvin scale starts at 0 K, which is absolute zero. This temperature is defined as the theoretical temperature at which all particle motion in matter stops. Absolute zero is –273.15°C. You can convert between the scales by adding or subtracting this value, which is often rounded to the nearest whole number.
![]()
Example:
What temperature on the Celsius scale is equivalent to a temperature of 365 K?
![]()
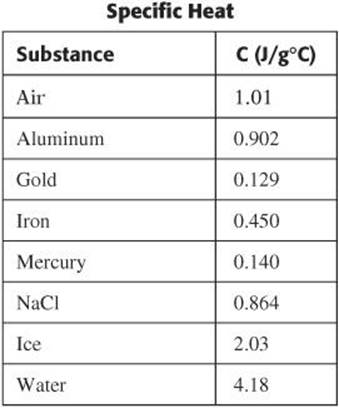
Specific Heat
The specific heat of a substance is the amount of heat required to raise the temperature of one mass unit by one degree Celsius. The following equation shows the relationship among the heat added to a substance Q, the specific heat c, the mass m, and the temperature T.
![]()
The equation holds true both for substances that absorb energy from their surroundings and those that transfer energy to their surroundings. When energy is absorbed, Q and ΔT are positive. When energy is transferred out of a substance, Q and ΔT are negative.
Water has one of the highest specific heats of any common substance at 4.186 J/g°C. This means that it takes 4.186 J of energy to raise the temperature of 1 gram of water by 1°C. It is this property that makes water extremely important in temperature regulation. The specific heat of copper is 0.385 J/g°C. A lot less heat is required to raise the temperature of one gram of copper by 1°C than it is to raise the temperature of water by the same amount. The table shows the specific heats of some common substances.
The term heat capacity is often used to describe a sample as a whole rather than as a unit mass. The heat capacity of a sample is the product of the mass of the sample and the specific heat of the material.
Example:
What is the specific heat of copper if 204.75 J of energy raises the temperature of 15 g of copper from 18°C to 53°C?
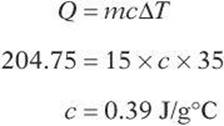
Phase Changes
It was stated earlier the temperature of a substance can rise if it absorbs heat and therefore increases its thermal energy. The reason for choosing the word can is that an increase in thermal energy does not always result in a temperature increase. During a phase change, the increased energy goes into overcoming the attractive forces between particles and, therefore, does not change the temperature. The heat involved in phase changes can be represented by the following equation, where L is the heat of transformation.
![]()
The value of L depends on the substance as well as the process. The heating curve for water is useful for reviewing the different processes.

Beginning toward the left of the graph, you find water in the solid state at –40°C. As heat is added, the temperature increases until it reaches 0°C, which is the melting point of water. At this temperature, any added heat causes the solid ice to change into liquid water. The flat portion of the graph represents the phase change. The amount of heat required for this change is known as the heat of fusion. For water, the heat of fusion is 334 J/g.
Once all of the water in the sample is liquid, adding heat will again raise the temperature. The temperature continues to rise until it reaches 100°C, which is the boiling point of water. At this temperature, any added heat causes the liquid water to change to water vapor (steam). The flat portion of the graph again represents the change of phase. The amount of heat required for this change is known as the heat of vaporization. For water, the heat of vaporization is 2260 J/g.
If you review the graph in reverse, you find the opposite processes. When heat is released at 100°C, the gas condenses into a liquid. When heat is released at 0°C, the liquid freezes into a solid.
Thermal Expansion in Solids
Now that you have reviewed general information about all phases, it would be helpful to consider some specific properties of certain phases of matter. For example, you may know that when a metal bridge heats up, it expands. This is the reason expansion joints are included in bridge construction. Most solids expand when heated and contract when cooled. (Water is an exception to the rule.)
The change in length L, known as linear expansion, is proportional to the original length Lo and the change in temperature T.
![]()
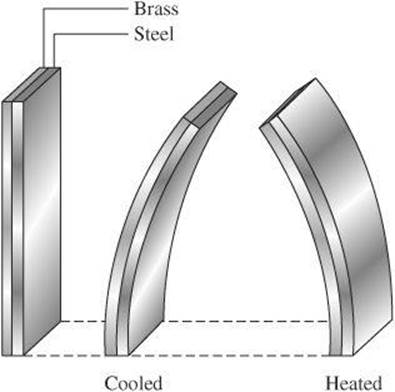
In this equation, α is the coefficient of linear expansion and depends on the material from which an object is made. When the same lengths of different materials experience the same temperature change, the thermal expansion will be different. For example, the coefficient of linear expansion for brass is 19 × 10–6/°C, which means that each centimeter of brass will increase in length by 19 × 10–6 cm for each increase of 1°C. The coefficient of linear expansion for steel is 11 × 10–6/°C. So, for the same temperature increase of 1°C, each centimeter of steel will increase in length by only 11 × 10–6 cm.
As you can see, the changes in length are quite small. The result is more dramatic, however, when two different kinds of metal are fused together. This is the principle behind a bimetallic strip, or compound bar. The strip is made by joining two different metals together, such as brass and steel. When heated, the brass expands more than the steel so the strip curves. The curving can cause the strip to either open or close an electric circuit, such as in a heating system. When cooled, the strip will bend in the opposite direction.
Example:
An engineer is working with a 2-m brass rod and a 1-m aluminum rod. The rods are each fixed at one end and have a gap of 2.0 × 10–3 m between them when the temperature is 25°C. What must the temperature be for the two rods to touch?
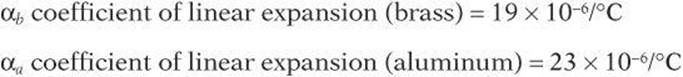
The rods will touch when they expand a length equal to the gap.
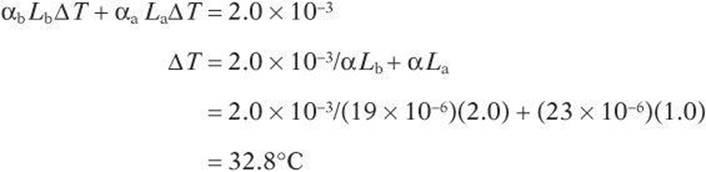
The original temperature was 25°C, so the rods will touch when the temperature rises to 57.8°C.
The Gas Laws
Several laws describe the relationships among the pressure, volume, and temperature of gases. According to Charles’ Law, volume is directly proportional to temperature if pressure is held constant. The relationship is summarized in the following equation. Note that the volume can be expressed in any units as long as the same units are used for V1 and V2.
![]()
According to Boyles’ Law, volume is inversely related to pressure if temperature is held constant. The relationship is summarized in the following equation. Again, note that the units can vary, but they must be the same for the initial and final values.
![]()
The two laws can be combined into a general gas law as shown here.
![]()
Test-Taking Hint
You will rarely be asked to memorize specific values for SAT Physics. However, some values come up often and may be helpful to know, such as absolute zero and the melting and boiling points of water.
REVIEW QUESTIONS
Select the choice that best answers the question or completes the statement.
1. A temperature of 90°C is equivalent to which of the following Kelvin temperatures?
(A) –183 K
(B) 17 K
(C) 190 K
(D) 363 K
(E) 413 K
2. What is absolute zero?
(A) the temperature on the Celsius scale at which water freezes
(B) the temperature at which the Celsius and Kelvin scales intersect
(C) the temperature at which heat energy is transferred from a substance
(D) the temperature at which molecular motion ceases
(E) the temperature on the Kelvin scale at which water boils
3. The temperature of a sample of matter is most closely associated with the
(A) average kinetic energy of the molecules.
(B) internal energy of the sample.
(C) total kinetic energy of the molecules.
(D) average potential energy of the sample.
(E) specific heat of the molecules.
4. A scientist adds 36 J of heat to 12 g of aluminum. By how much will the temperature increase? The specific heat of aluminum is 0.902 J/g°C.
(A) 0.37 J
(B) 1.08 J
(C) 2.43 J
(D) 3.33 J
(E) 4.79 J
5. How much heat is lost when a sample of aluminum with a mass of 16.231 g cools from 320.0°C to 52.0°C? The specific heat of aluminum is 0.902 J/g°C.
(A) 1.8 × 102 J
(B) 3.9 × 103 J
(C) 4.3 × 103 J
(D) 5.5 × 103 J
(E) 1.5 × 104 J
6. How much energy is needed to raise the temperature of 71.0 g of water from 24.0 to 59.0°C? The specific heat of water = 4.184 J/g°C.
(A) 7.1 × 103 J
(B) 1.0 × 104 J
(C) 1.8 × 104 J
(D) 2.4 × 104 J
(E) 9.9 × 105 J
7. Engineers constructed a steel bridge with a length of 2000 m. If the coefficient of linear expansion for steel is 1.0 × 10–5/°C, how much expansion should the engineers expect if the temperature rises from 10°C to 30°C?
(A) 0.4 m
(B) 0.6 m
(C) 1.0 m
(D) 1.2 m
(E) 1.4 m
8. The volume of an ideal gas is doubled without changing the temperature. What will happen to the pressure of the gas?
(A) it will be reduced by ¼.
(B) it will be halved.
(C) it will stay the same.
(D) it will be doubled.
(E) it will be quadrupled.
Questions 9 and 10 relate to the diagram below, which shows the heating curve for water.
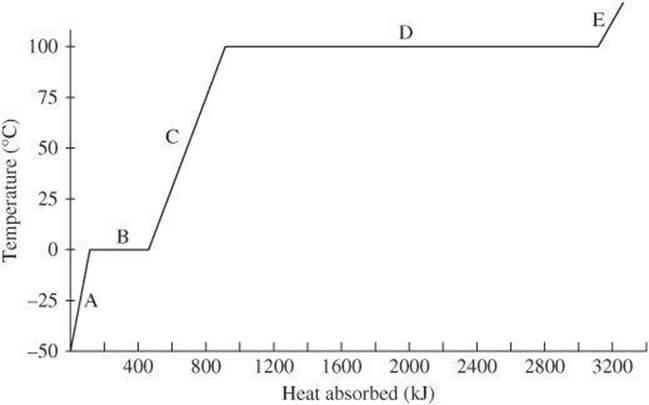
9. Which part of the graph represents the heat of vaporization?
(A) A
(B) B
(C) C
(D) D
(E) E
10. Why is section B of the graph shorter than section D?
(A) the volume of the solid is less than the volume of the liquid.
(B) the pressure at which water melts is less than the pressure at which it boils.
(C) less water is destroyed during melting than during boiling.
(D) melting occurs more quickly than boiling.
(E) less heat is required to melt the solid than to boil the liquid.
QUESTION ANSWERS AND EXPLANATIONS
1. ![]()
2. D Absolute zero, which is the starting point of the Kelvin scale, is a theoretical temperature at which molecular motion would stop.
3. A The temperature of a sample is the average kinetic energy of the molecules in the sample.
4. ![]() , so
, so ![]() , so
, so ![]() .
.
5. ![]()
6. ![]()
7. ![]()
8. ![]() and
and ![]() , so
, so ![]() . Therefore,
. Therefore, ![]()
9. D The heat of vaporization is the amount of heat required to change the liquid to the gas. It is represented by the flat portion of the graph at the boiling point of water.
10. E The lines on the graph relate the amount of heat to the temperature. The flat portions indicate changes of phase, during which temperature remains constant. The length of the flat portions is proportional to the amount of heat required to change the phase of a sample of water.